NSW Y12 Maths - Extension 1 Differential Equations Solving y'=f(x)

Solving y=f(x) Videos How to buy Buy Books

Resources for Solving y'=f(x)

Questions

17
With Worked Solution

Click Here
Video Tutorials

1

Click Here

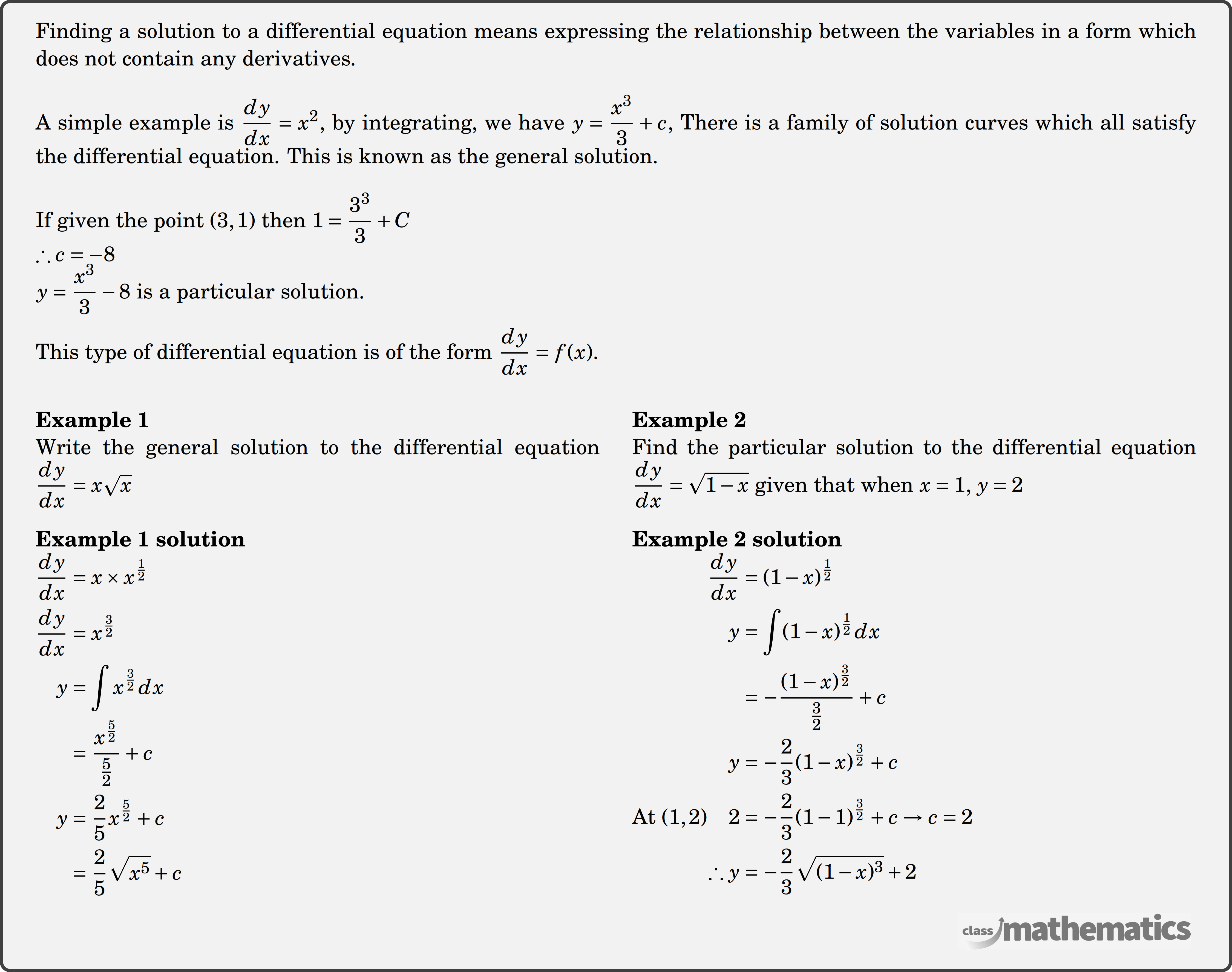
Solving y'=f(x) Theory

$Finding a solution to a differential equation means expressing the relationship between the variables in a form which does not contain any derivatives. \\ A simple example is $\dfrac{d y}{d x}=x^2$, by integrating, we have $y=\dfrac{x^3}{3}+c$, There is a family of solution curves which all satisfy the differential equation. This is known as the general solution. \\ If given the point $(3,1)$ then $1=\dfrac{3^3}{3}+C$\\ $\therefore c=-8$\\ $y=\dfrac{x^3}{3}-8$ is a particular solution.\\ This type of differential equation is of the form $\dfrac{d y}{d x}=f(x)$.\\ \begin{multicols}{2} \textbf{Example 1}\\%question 118326 Write the general solution to the differential equation $\dfrac{dy}{dx}=x \sqrt{x}$\\ \textbf{Example 1 solution}\\ $\begin{aligned} \frac{d y}{d x} &=x \times x^{\frac{1}{2}} \\ \frac{d y}{d x} &=x^{\frac{3}{2}} \\ y &=\int x^{\frac{3}{2}} d x \\ &=\frac{x^{\frac{5}{2}}}{\frac{5}{2}}+c \\ y &=\frac{2}{5} x^{\frac{5}{2}}+c \\ &=\frac{2}{5} \sqrt{x^5}+c \end{aligned}$\\ \columnbreak \textbf{Example 2}\\%question 118328 Find the particular solution to the differential equation $\dfrac{dy}{dx}=\sqrt{1-x}$ given that when $x=1, \, y=2$ \\ \textbf{Example 2 solution}\\ $\begin{aligned} \frac{d y}{d x} &=(1-x)^{\frac{1}{2}} \\ y &=\int(1-x)^{\frac{1}{2}} d x \\ &=-\frac{(1-x)^{\frac{3}{2}}}{\frac{3}{2}}+c \\ y &=-\frac{2}{3}(1-x)^{\frac{3}{2}}+c \\ \text{At } (1,2) \quad 2 &=-\frac{2}{3}(1-1)^{\frac{3}{2}}+c \rightarrow c=2 \\ \therefore y &=-\frac{2}{3} \sqrt{(1-x)^3}+2 \end{aligned}$\\ \end{multicols}$

Create account

I am..

A teacher
A student
A tutor

Please enter your details

First Name.

Last Name

Email Address

State

I agree with your terms of service

Back to Topic (Differential Equations)

Videos

Videos relating to Solving y'=f(x).

Solving y=f(x) - Video - Solving y'=f(x)

You must be logged in to access this resource

Plans & Pricing

With all subscriptions, you will receive the below benefits and unlock all answers and fully worked solutions.

Teachers Tutors

Features

Free

Pro

All Content

All courses, all topics

Questions

Answers

Worked Solutions

System

Your own personal portal

Quizbuilder

Class Results

Student Results

Exam Revision

Revision by Topic

Practise Exams

Answers

Worked Solutions
Awesome Students

Features

Free

Pro

Content

Any course, any topic

Questions

Answers

Worked Solutions

Extended Response

Questions and Answers

Fully Worked Solutions

Videos

Curated Topic Videos

Premium Topic Videos

System

Your own personal portal

Basic Results

Analytics

Study Recommendations

Exam Revision

Revision by Topic

Practise Exams

Answers

Worked Solutions