Homepage

Course Index

Build a Quiz

Subscriptions

Courses

NSW Y12 Maths Standard 1 NSW Y12 Maths Standard 2 NSW Y12 Maths Advanced NSW Y12 Maths Extension 1 NSW Y12 Maths Extension 2

Topics

Trig Equations Proof Vectors Calculus Differential Equations Projectile Motion Binomial Distribution

NSW Y12 Maths - Extension 1 Binomial Distribution Normal Approximation of the Sample Proportion

Resources for Normal Approximation of the Sample Proportion

  • Questions

    10

    With Worked Solution
    Click Here
  • Video Tutorials

    1


    Click Here
  • HSC Questions

    4

    With Worked Solution
    Click Here

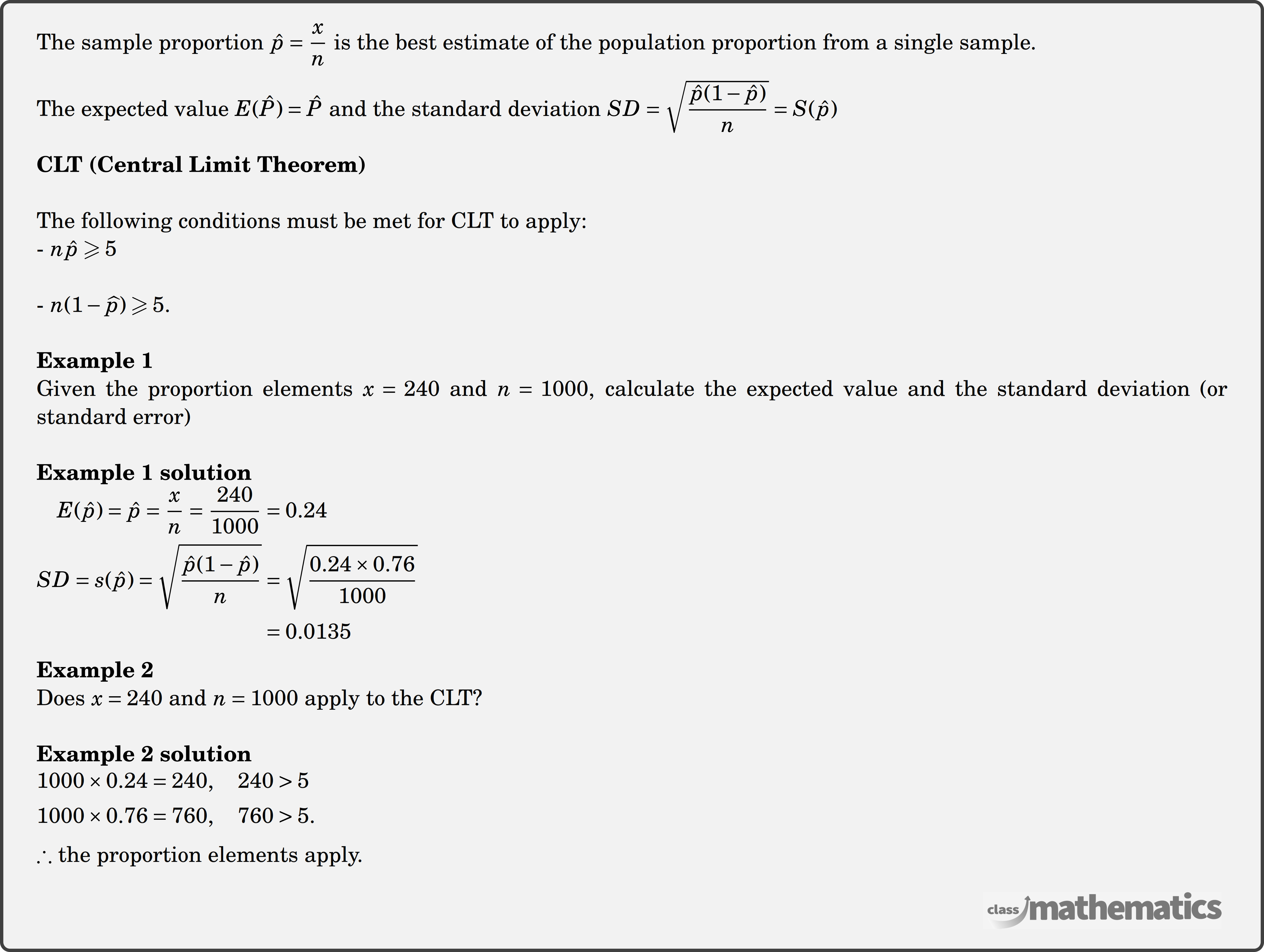
Normal Approximation of the Sample Proportion Theory

The sample proportion \(\hat{p}=\dfrac{x}{n}\) is the best estimate of the population proportion from a single sample.\\ The expected value \(E(\hat{P})=\hat{P}\) and the standard deviation \(S D=\sqrt{\dfrac{\hat{p}(1-\hat{p})}{n}}=S(\hat{p})\)\\ \textbf{CLT (Central Limit Theorem)}\\ The following conditions must be met for CLT to apply:\\ - \(n \hat{p} \geqslant 5\)\\ - \(n(1-\widehat{p}) \geqslant 5\).\\ \textbf{Example 1}\\ Given the proportion elements \(x=240\) and \(n=1000\), calculate the expected value and the standard deviation (or standard error)\\ \textbf{Example 1 solution}\\ $\begin{aligned} E(\hat{p})=\hat{p}=\frac{x}{n}=\frac{240}{1000} & =0.24 \\ SD=s(\hat{p})=\sqrt{\frac{\hat{p}(1-\hat{p})}{n}} & =\sqrt{\frac{0.24 \times 0.76}{1000}} \\ & =0.0135 \end{aligned}$\\ \textbf{Example 2}\\ Does \(x=240\) and \(n=1000\) apply to the CLT?\\ \textbf{Example 2 solution}\\ $\begin{aligned} 1000 \times 0.24 & =240,\quad 240>5 \\ 1000 \times 0.76 & =760,\quad 760>5 . \end{aligned}$\\ \(\therefore\) the proportion elements apply.\\

Create account

Access content straight away with a two week free trial

I am..

Please enter your details

I agree with your terms of service




Videos

Videos relating to Normal Approximation of the Sample Proportion.

  • Normal Approximation of the Sample Proportion - Video - Normal Approximation for the Sample Proportion

    You must be logged in to access this resource

Plans & Pricing

With all subscriptions, you will receive the below benefits and unlock all answers and fully worked solutions.

  • Teachers Tutors
    Features
    Free
    Pro
    All Content
    All courses, all topics
     
    Questions
     
    Answers
     
    Worked Solutions
    System
    Your own personal portal
     
    Quizbuilder
     
    Class Results
     
    Student Results
    Exam Revision
    Revision by Topic
     
    Practise Exams
     
    Answers
     
    Worked Solutions
  • Awesome Students
    Features
    Free
    Pro
    Content
    Any course, any topic
     
    Questions
     
    Answers
     
    Worked Solutions
    System
    Your own personal portal
     
    Basic Results
     
    Analytics
     
    Study Recommendations
    Exam Revision
    Revision by Topic
     
    Practise Exams
     
    Answers
     
    Worked Solutions