NSW Y11 Maths - Extension 1 Polynomials Remainder Theorem

Resources for Remainder Theorem

• Questions

  32

  With Worked Solution
  Click Here
• Video Tutorials

  3

  
  
  Click Here
• HSC Questions

  4

  With Worked Solution
  Click Here

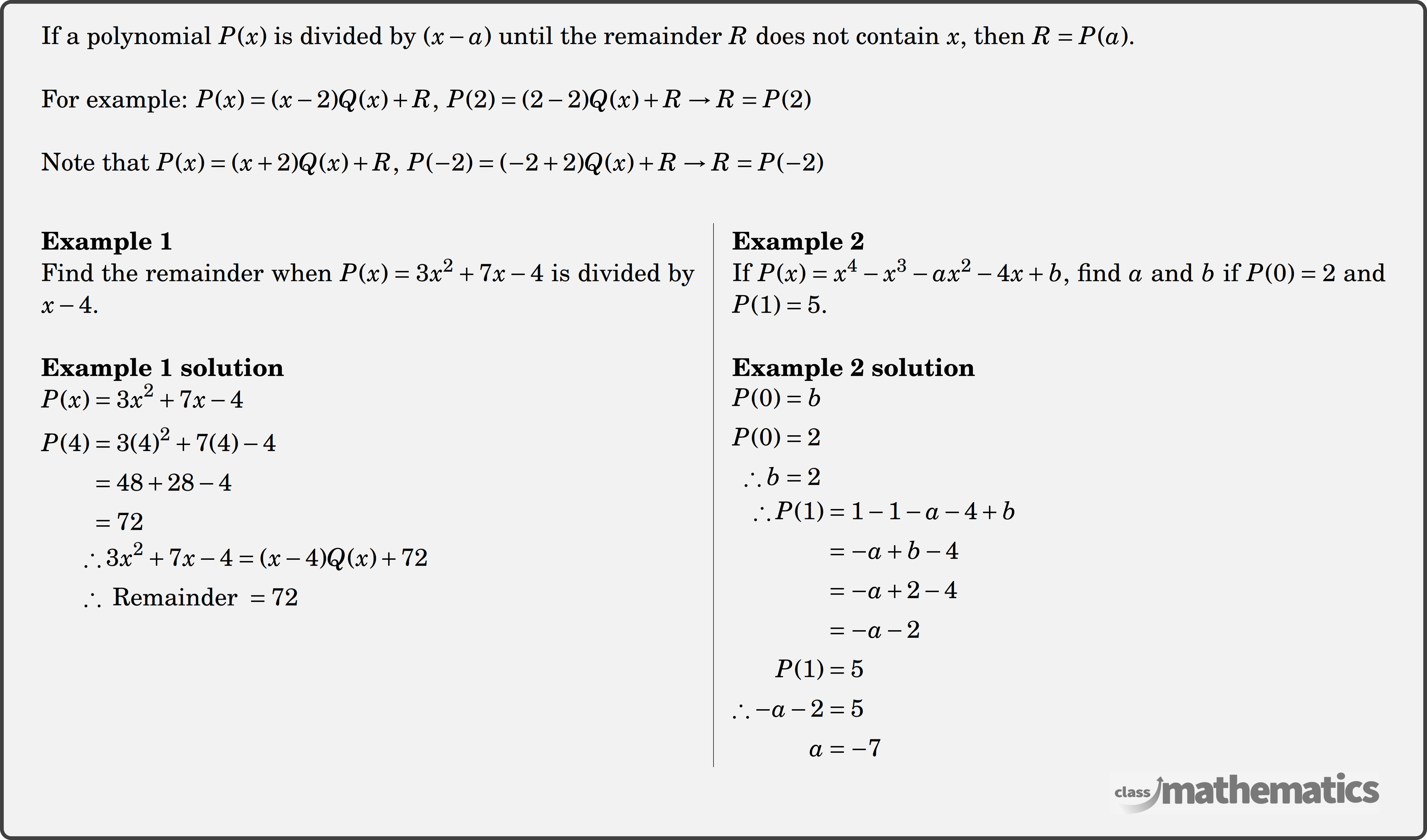
Remainder Theorem Theory

NSW Syllabus Reference

NSW Syllabus Reference: ME-F2.1: Remainder and factor theorems. This will require student to 

• define a general polynomial in one variable, \(x\), of degree \(n\) with real coefficients to be the expression: \(a_{n}x^n+a_{n-1}x^{n-1}+\cdots +a_{2}x^{2}+a_{1}x+a_{0}\), where \(a_{n}\neq 0\)
• use division of polynomials to express \(P(x)\) in the form \(P(x)=A(x).Q(x)+R(x)\) where \(\deg R(x)<\deg A(x)\) and \(A(x) \) is a linear or quadratic divisor, \(Q(x)\) the quotient and \(R(x)\) the remainder
• prove and apply the factor theorem and the remainder theorem for polynomials and hence solve simple polynomial equations (ACMSM089, ACMSM091)

Ref: https://educationstandards.nsw.edu.au/