NSW Y11 Maths - Extension 1 Inverse Trig Functions and Identities Trig Products as Sums or Differences

Resources for Trig Products as Sums or Differences

• Questions

  28

  With Worked Solution
  Click Here
• Video Tutorials

  1

  
  
  Click Here

Trig Products as Sums or Differences Theory

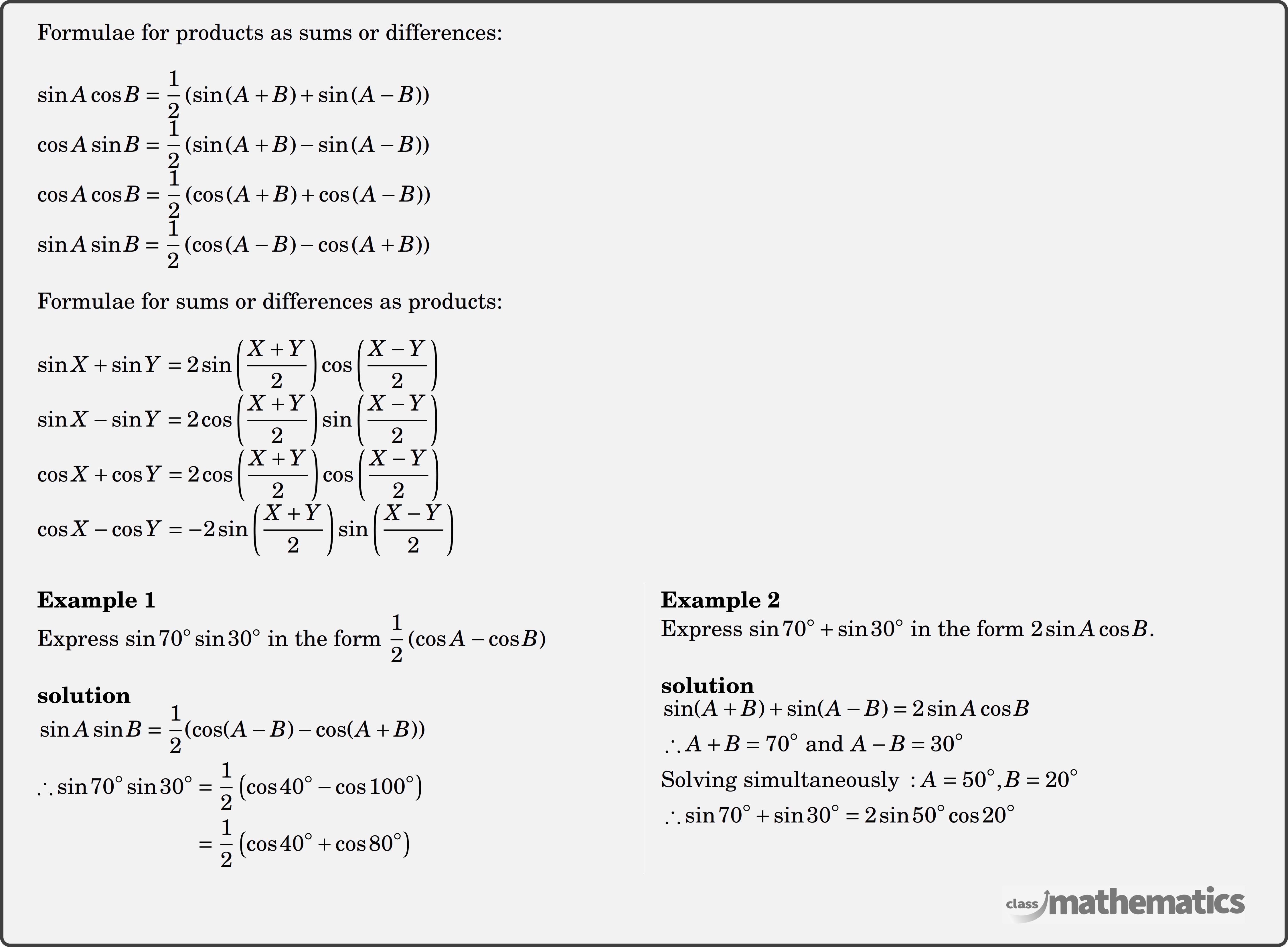
NSW Syllabus Reference

NSW Syllabus Reference: ME-T2 Further Trigonometric Identities. This will require student to 

• derive and use the sum and difference expansions for the trigonometric functions $\sin (𝐴\pm 𝐵),\cos (𝐴\pm 𝐵)$ and $\tan (𝐴\pm 𝐵)$ (ACMSM044)
• derive and use the double angle formulae for $\sin 2𝐴,\cos 2𝐴$ and $\tan 2𝐴$ (ACMSM044)
• derive and use expressions for $\sin 𝐴,\cos 𝐴$ and $\tan 𝐴$ in terms of $t$ where $t=\tan {\displaystyle \frac{A}{2}}$ (the $t$-formulae)
• derive and use the formulae for trigonometric products as sums and differences for $\cos 𝐴cos𝐵,sin𝐴sin𝐵,sin𝐴cos𝐵$ and $cos𝐴sin𝐵$ (ACMSM047)

Ref: https://educationstandards.nsw.edu.au/