7
With Worked Solution1
1
With Worked Solution
Videos relating to Graphing-Adding Ordinates.
With all subscriptions, you will receive the below benefits and unlock all answers and fully worked solutions.
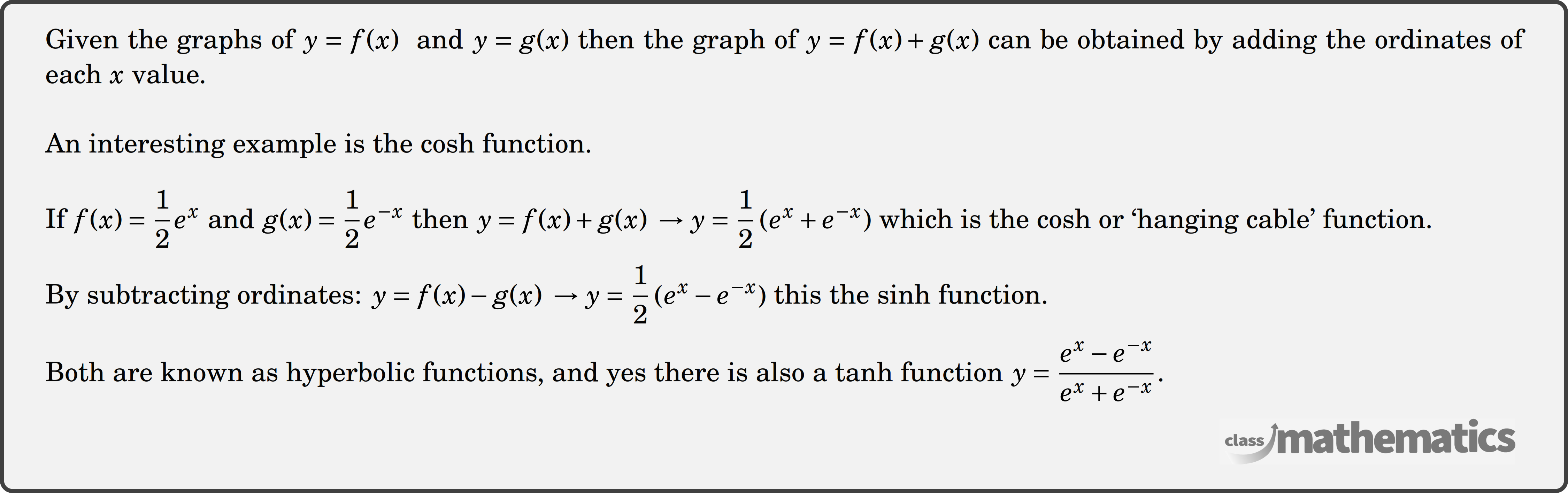
Given the graphs of \(y = f(x)\) and \(y = g(x)\) then the graph of \(y = f(x) + g(x)\) can be obtained by adding the ordinates of each \(x\) value.
An interesting example is the cosh function.
If \(f(x) = \dfrac{1}{2}{e^x}\) and \(g(x) = \dfrac{1}{2}{e^{ - x}}\) then \(y = f(x) + g(x)\,\, \to y = \dfrac{1}{2}\left( {{e^x} + {e^{ - x}}} \right)\) which is the cosh or ‘hanging cable’ function.
By subtracting ordinates: \(y = f(x) - g(x)\,\, \to y = \dfrac{1}{2}\left( {{e^x} - {e^{ - x}}} \right)\) this the \(sinh\) function.
Both are known as hyperbolic functions, and yes there is also a \(tanh\) function \(y = \dfrac{{{e^x} - {e^{ - x}}}}{{{e^x} + {e^{ - x}}}}\).
NSW Syllabus Reference: ME-F1.1: Graphical relationships. This will require student to
